Factors of 130 | How to Find the Factors of 130 🔢

Table Of Contents

Table of Contents

108 Learners

Last updated on December 2nd, 2024

Factors of 130

Foundation

Intermediate

Advance Topics

Factors of any number are the dividers or multipliers that can divide the number fully and can be multiplied together to produce the given product, 130. Do you know, factors form the basic approach to solve some general mathematical procedures? This article will give you the insights of factors of 130.

What are the Factors of 130?

The factors of 130 or the numbers which divide 130 exactly are:

1,2,5,10,13,26,65, and 130.

Negative factors of 130: -1,-2,-5,-10,-13,-26,-65,-130.

Prime factors of 130: 2,5,13

Prime factorization of 130: 2×5×13

The sum of factors of 130:

1+2+5+10+13+26+65+130= 252

How to Find the Factors of 130

For finding factors of 130, we will be learning these below-mentioned methods:

Multiplication Method

Division Method

Prime Factor and Prime Factorization

Factor Tree

Finding Factors using Multiplication Methods

This particular method often finds the pair of factors which, on multiplication together, produces 130. Let us find the pairs which, on multiplication, yields 130.

1×130=130

2×65=130

5×26=130

10×13=130

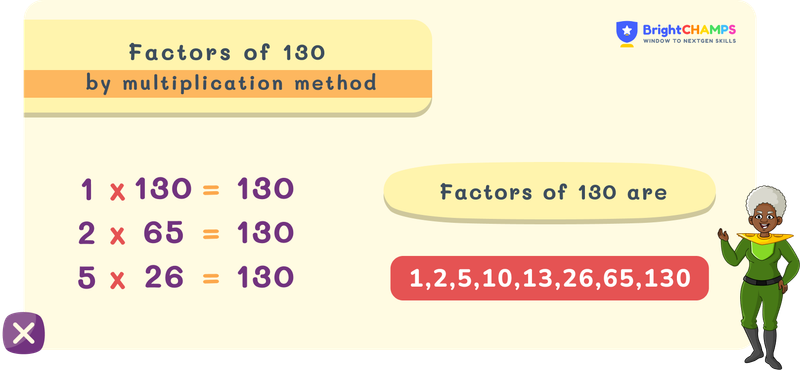
So, factors of 130 are: 1,2,5,10,13,26,65, and 130.

Finding Factors using Division Method

The division method finds the factors that evenly divides the given number 130. In this process, we have to divide 130 by all possible natural numbers less than 130 and check.

1,2,5,10,13,26,65, and 130 are the only factors that the number 130 has. So to verify the factors of 130 using the division method, we just need to divide 130 by each factor.

130/1 =130

130/2=65

130/5=26

130/10=13

130/13=10

130/26=5

130/65=2

130/130=1

Prime Factors and Prime Factorization

Prime Factorization is the easiest process to find prime factors. It decomposes 130 into a product of its prime integers.

Prime Factors of 130: 2,5,13.

Prime Factorization of 130: 2×5×13

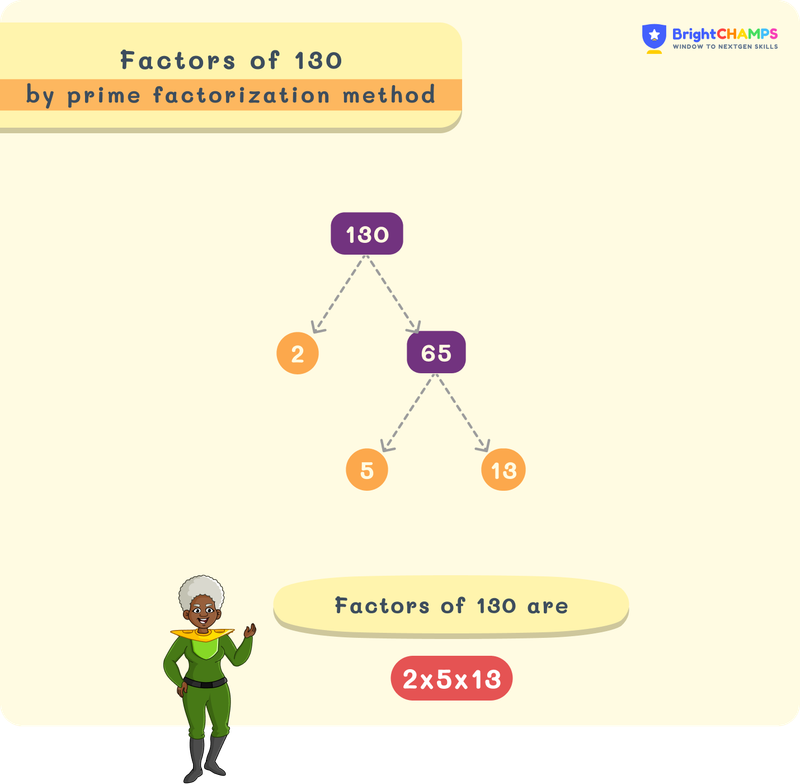
Factor tree

The number 130 is written on top and two branches are extended.

Fill in those branches with a factor pair of the number above, i.e., 130.

Continue this process until each branch ends with a prime factor (number).

The first two branches of the factor tree of 130 are 2 and 65, then proceeding to 65, we get 5 and 13. So, now the factor tree for 130 is achieved.

Factor Pairs

Positive pair factors: (1,130), (2,65), (5,26), (10,13).

Negative pair factors: (-1,-130), (-2,-65), (-5,-26), (-10,-13).

Important Glossaries for Factors of 130

Multipliers - Number which multiplies or a number by which another number is multiplied.

Dividers - A number that divides.

Prime Factorization - It involves factoring the number into its prime factors.

Prime factors - These are the prime numbers which on multiplication together results into the original number whose prime factors are to be obtained.

Composite numbers - These are numbers having more than two factors.

Multiple - It is a product of the given number and any other integer.