Summarize this article:
 176 Learners
176 LearnersLast updated on August 5, 2025
Square Root of 393

If a number is multiplied by the same number, the result is a square. The inverse of the square is a square root. The square root is used in various fields such as engineering, finance, etc. Here, we will discuss the square root of 393.

What is the Square Root of 393?
The square root is the inverse of the square of a number. 393 is not a perfect square. The square root of 393 is expressed in both radical and exponential form. In the radical form, it is expressed as √393, whereas in exponential form it is (393)^(1/2). √393 ≈ 19.823, which is an irrational number because it cannot be expressed in the form of p/q, where p and q are integers and q ≠ 0.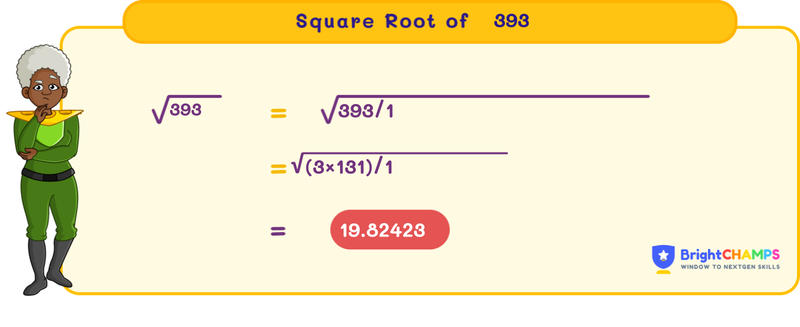

Finding the Square Root of 393
The prime factorization method is used for perfect square numbers. However, for non-perfect square numbers, the long division method and approximation method are used. Let us now learn the following methods:
- Prime factorization method
- Long division method
- Approximation method

Square Root of 393 by Prime Factorization Method
The product of prime factors is the prime factorization of a number. Now, let us look at how 393 is broken down into its prime factors.
Step 1: Finding the prime factors of 393 Breaking it down, we get 3 × 131: 3^1 × 131^1
Step 2: Now that we found the prime factors of 393, the second step is to make pairs of those prime factors. Since 393 is not a perfect square, the digits of the number can’t be grouped into pairs.
Therefore, calculating √393 using prime factorization is not straightforward.

Square Root of 393 by Long Division Method
The long division method is particularly used for non-perfect square numbers. In this method, we should check the closest perfect square number for the given number. Let us now learn how to find the square root using the long division method, step by step.
Step 1: To begin with, we need to group the numbers from right to left. In the case of 393, we need to group it as 93 and 3.
Step 2: Now we need to find n whose square is less than or equal to 3. We can say n is ‘1’ because 1 × 1 is less than or equal to 3. Now the quotient is 1, and after subtracting 1, the remainder is 2.
Step 3: Now let us bring down 93, making the new dividend 293. Add the old divisor with the same number, 1 + 1, which gives us 2 as the new divisor.
Step 4: The new divisor will be the sum of the dividend and quotient. Now we get 2n as the new divisor, and we need to find the value of n.
Step 5: The next step is finding 2n × n ≤ 293. Let us consider n as 9, now 29 × 9 = 261.
Step 6: Subtract 261 from 293, and the difference is 32. The quotient is 19.
Step 7: Since the dividend is less than the divisor, we need to add a decimal point. Adding the decimal point allows us to add two zeroes to the dividend. Now the new dividend is 3200.
Step 8: Now we need to find the new divisor, which is 398, because 398 × 8 = 3184.
Step 9: Subtracting 3184 from 3200, we get the result 16.
Step 10: Now the quotient is 19.8.
Step 11: Continue doing these steps until we get two numbers after the decimal point.
So the square root of √393 ≈ 19.82.

Square Root of 393 by Approximation Method
The approximation method is another method for finding square roots. It is an easy method to find the square root of a given number. Now let us learn how to find the square root of 393 using the approximation method.
Step 1: Now we have to find the closest perfect square of √393.
The smallest perfect square less than 393 is 361, and the largest perfect square greater than 393 is 400.
√393 falls somewhere between 19 and 20.
Step 2: Now we need to apply the formula:
(Given number - smallest perfect square) ÷ (Greater perfect square - smallest perfect square)
Using the formula: (393 - 361) ÷ (400 - 361) = 32 ÷ 39 ≈ 0.82
Adding the decimal value to the lower perfect square root: 19 + 0.82 = 19.82, so the square root of 393 is approximately 19.82.

Common Mistakes and How to Avoid Them in the Square Root of 393
Students often make mistakes while finding the square root, such as forgetting about the negative square root, skipping steps in the long division method, etc. Now, let us look at a few of these mistakes in detail.

Square Root of 393 Examples

Problem 1
Can you help Max find the area of a square box if its side length is given as √193?

The area of the square is approximately 193 square units.
Explanation
The area of the square = side².
The side length is given as √193
Area of the square = side² = √193 × √193 ≈ 13.89 × 13.89 ≈ 193
Therefore, the area of the square box is approximately 193 square units.

Problem 2
A square-shaped building measuring 393 square feet is built; if each of the sides is √393, what will be the square feet of half of the building?

196.5 square feet
Explanation
We can just divide the given area by 2 as the building is square-shaped.
Dividing 393 by 2 = 196.5
So half of the building measures 196.5 square feet.

Problem 3
Calculate √393 × 5.

99.115
Explanation
The first step is to find the square root of 393, which is approximately 19.823.
The second step is to multiply 19.823 by 5.
So 19.823 × 5 ≈ 99.115.

Problem 4
What will be the square root of (193 + 7)?

The square root is approximately 14.
Explanation
To find the square root, we need to find the sum of (193 + 7). 193 + 7 = 200, and then √200 ≈ 14.142.
Therefore, the square root of (193 + 7) is approximately 14.142.

Problem 5
Find the perimeter of the rectangle if its length ‘l’ is √193 units and the width ‘w’ is 38 units.

We find the perimeter of the rectangle as approximately 103.78 units.
Explanation
Perimeter of the rectangle = 2 × (length + width)
Perimeter = 2 × (√193 + 38) ≈ 2 × (13.89 + 38) ≈ 2 × 51.89 = 103.78 units.


FAQ on Square Root of 393
1.What is √393 in its simplest form?
2.Mention the factors of 393.
3.Calculate the square of 393.
4.Is 393 a prime number?
5.393 is divisible by?
6.How does learning Algebra help students in United States make better decisions in daily life?
7.How do technology and digital tools in United States support learning Algebra and Square Root of 393?
8.How can cultural or local activities in United States support learning Algebra topics such as Square Root of 393?
9.Does learning Algebra support future career opportunities for students in United States?

Important Glossaries for the Square Root of 393
- Square root: A square root is the inverse of a square. Example: 4² = 16, and the inverse of the square is the square root, that is, √16 = 4.
- Irrational number: An irrational number is a number that cannot be written in the form of p/q, where q is not equal to zero and p and q are integers.
- Principal square root: A number has both positive and negative square roots; however, it is usually the positive square root that is used in real-world applications. This is known as the principal square root.
- Non-perfect square: A non-perfect square is a number that does not have an integer as its square root.
- Radical form: Radical form expresses the square root of a number using the symbol √.

Explore More algebra
![Important Math Links Icon]() Previous to Square Root of 393
Previous to Square Root of 393
![Important Math Links Icon]() Next to Square Root of 393
Next to Square Root of 393


Jaskaran Singh Saluja
About the Author
Jaskaran Singh Saluja is a math wizard with nearly three years of experience as a math teacher. His expertise is in algebra, so he can make algebra classes interesting by turning tricky equations into simple puzzles.
Fun Fact
: He loves to play the quiz with kids through algebra to make kids love it.




