107 LearnersLast updated on May 26th, 2025
Square Root of -119
The square root of a number is a value that, when multiplied by itself, gives the original number. However, when dealing with negative numbers, their square roots are not real numbers. This concept is used in various fields such as engineering, physics, and complex number theory. Here, we will discuss the square root of -119.
What is the Square Root of -119?
The square root of a negative number, such as -119, is not a real number. Instead, it is an imaginary number. The square root of -119 can be expressed in terms of the imaginary unit 'i', where i is the square root of -1. Therefore, the square root of -119 is expressed as √(-119) = √119 * i = 10.9087i, which is an imaginary number.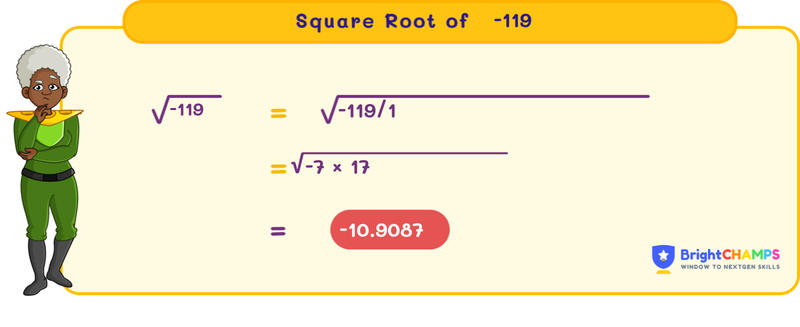
Understanding the Square Root of -119
To find the square root of a negative number, we use the concept of imaginary numbers. Let us now learn:
1. Expressing negative square roots in terms of 'i'
2. Calculating the magnitude of the imaginary number
Expressing Negative Square Roots in Terms of 'i'
The imaginary unit 'i' is defined as √-1. Therefore, the square root of any negative number can be expressed using 'i'. For example, the square root of -119 can be written as: √(-119) = √119 * √(-1) = √119 * i
Calculating the Magnitude of the Imaginary Number
To find the magnitude of the square root of -119, we calculate the square root of the positive component 119:
Step 1: Find the square root of 119, which is approximately 10.9087.
Step 2: Combine it with 'i': √(-119) = 10.9087i
Applications of Imaginary Numbers
Imaginary numbers are used in various fields such as:
1. Electrical engineering for analyzing AC circuits
2. Control systems for representing phase differences
3. Signal processing for Fourier transforms
Common Mistakes and How to Avoid Them in Understanding the Square Root of -119
When working with negative square roots, students can make mistakes by incorrectly treating them as real numbers or by mishandling the imaginary unit. Let us explore some common mistakes and how to avoid them.
Mistake 1
Treating Negative Square Roots as Real Numbers
It is crucial to understand that the square root of a negative number is not a real number. Forgetting to include the imaginary unit 'i' is a common mistake.
For example, √(-4) should be written as 2i, not as 2.
Square Root of -119 Examples
Problem 1
If Max encounters an electrical circuit problem involving √(-119), how should he interpret it?
Max should interpret √(-119) as an imaginary number.
Explanation
In electrical engineering, imaginary numbers like √(-119) = 10.9087i are used to represent certain quantities in AC circuits involving phase shifts or impedance.
Problem 2
In mathematics, why is √(-119) not considered a real number?
Because the square root of a negative number involves imaginary numbers.
Explanation
The square root of a negative number, such as -119, cannot be found on the real number line. Instead, it is represented as an imaginary number using 'i', making it √(-119) = 10.9087i.
Problem 3
How would √(-119) be used in signal processing?
As part of complex numbers in Fourier transforms.
Explanation
In signal processing, imaginary numbers like √(-119) = 10.9087i are used in Fourier transforms to analyze frequency components of signals.
Problem 4
What is the square of √(-119)?
The square is -119.
Explanation
When you square √(-119), you cancel out the square root, and the result is the original negative number: (√(-119))² = -119.
Problem 5
If the length of a side of a square is √(-119), what is the area?
The area is not a real number.
Explanation
Since the side length is √(-119) = 10.9087i, a complex number, the area (side²) is also not a real number and involves imaginary components.
FAQ on Square Root of -119
1.What is √(-119) in its simplest form?
2.How do you express the square root of a negative number?
3.What are imaginary numbers?
4.What is the principal square root of -119?
5.Can the square root of -119 be used in real-world applications?
Important Glossaries for the Square Root of -119
- Square root: The square root of a number is a value that, when multiplied by itself, gives the original number. For negative numbers, it involves imaginary numbers.
- Imaginary unit: Represented by 'i', it is defined as √-1. It is used to express the square roots of negative numbers.
- Imaginary number: A number that can be written as a real number multiplied by the imaginary unit 'i'. For example, 3i, where 3 is the magnitude.
- Complex number: A number that has both a real part and an imaginary part, such as 4 + 5i.
- Magnitude: The size or length of a vector or complex number, found by taking the square root of the sum of the squares of its components.
Explore More algebra
Previous to Square Root of -119
Jaskaran Singh Saluja
About the Author
Jaskaran Singh Saluja is a math wizard with nearly three years of experience as a math teacher. His expertise is in algebra, so he can make algebra classes interesting by turning tricky equations into simple puzzles.
Fun Fact
: He loves to play the quiz with kids through algebra to make kids love it.
