118 LearnersLast updated on May 26th, 2025
Square Root of -124
The square root is the inverse operation to squaring a number. When dealing with negative numbers, the square root introduces imaginary numbers, which are widely used in fields like engineering and complex analysis. Here, we will discuss the square root of -124.
What is the Square Root of -124?
The square root of a negative number involves the imaginary unit 'i', where i² = -1. Thus, the square root of -124 is expressed as √-124 = √124 * i. In its simplest form, √124 is approximated, and the complete expression becomes √124 * i ≈ 11.1355i, making it an imaginary number.
Understanding the Square Root of -124
When dealing with negative numbers under the square root, the result is not a real number but an imaginary number. The common methods for calculating square roots, such as the long division method or approximation, focus on the positive part of the number. For -124, we first find the square root of 124 and then multiply by 'i'.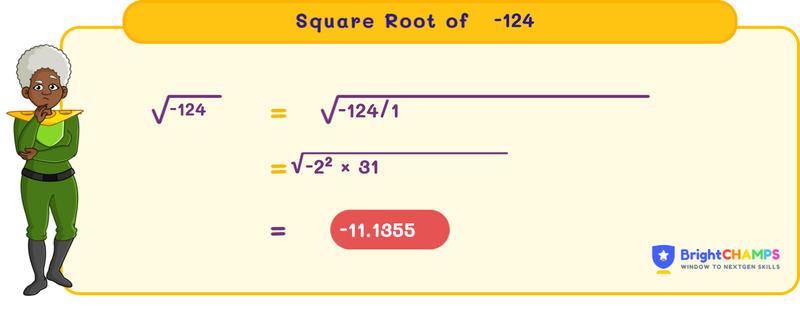
Calculating the Square Root of 124
To find the square root of 124, which is the positive component of -124, we can use methods like prime factorization or approximation:
Step 1: Prime factorization of 124: 2 x 2 x 31.
Step 2: Since 124 is not a perfect square, we approximate: √124 ≈ 11.1355.
The square root of -124 is then √124 * i ≈ 11.1355i.
Square Root of -124 using Imaginary Numbers
Imaginary numbers are used to handle the square roots of negative numbers. The formula i = √-1 is applied here:
Step 1: Calculate √124 ≈ 11.1355.
Step 2: Multiply by 'i' for the imaginary part: √-124 = 11.1355i.
Approximation Method for Square Root of 124
To approximate √124, we identify two perfect squares it is between:
Step 1: The closest perfect squares are 121 (11²) and 144 (12²).
Step 2: √124 is between 11 and 12. Using linear interpolation: (124 - 121) / (144 - 121) = 3 / 23 ≈ 0.1304, thus √124 ≈ 11 + 0.1304 = 11.1304.
Complex Numbers and the Square Root of -124
Complex numbers are formed by a real part and an imaginary part. The square root of -124 is entirely imaginary: √-124 = 11.1355i, with no real component.
Common Mistakes and How to Avoid Them in the Square Root of -124
Students may make errors when dealing with negative square roots, especially regarding the use of imaginary numbers. It's crucial to apply the concept of 'i' correctly. Here are some common mistakes and how to avoid them.
Mistake 1
Ignoring the Imaginary Unit
A frequent mistake is omitting the imaginary unit 'i' when finding the square root of a negative number. Remember that √-124 = 11.1355i, not a real number.
Square Root of -124 Examples
Problem 1
If the side length of a square is √-124, what is the area of the square?
The area is -124 square units.
Explanation
The area of a square = side².
If side = √-124, then side² = -124, resulting in an area of -124 square units, which is a conceptual representation in complex numbers.
Problem 2
Calculate the product of 2 and the square root of -124.
The result is approximately 22.271i.
Explanation
First, find the square root of -124: √-124 = 11.1355i.
Multiply by 2: 2 * 11.1355i = 22.271i.
Problem 3
What is the square of the square root of -124?
The square is -124.
Explanation
The square of √-124 = (-124), as squaring the square root returns the original negative number.
Problem 4
Find the result of dividing the square root of -124 by 2.
The result is approximately 5.5675i.
Explanation
Divide the imaginary square root by 2: √-124 = 11.1355i, so 11.1355i / 2 = 5.5675i.
Problem 5
What is the sum of √-124 and 5i?
The sum is approximately 16.1355i.
Explanation
Add the imaginary components directly: √-124 = 11.1355i, and 11.1355i + 5i = 16.1355i.
FAQ on Square Root of -124
1.What is the square root of -124?
2.Can the square root of a negative number be real?
3.How do you express the square root of -124 in terms of 'i'?
4.What is the principal square root of -124?
5.Is -124 a perfect square?
Important Glossaries for the Square Root of -124
- Imaginary number: A number that can be written as a real number multiplied by the imaginary unit 'i', which satisfies i² = -1.
- Complex number: A number that has both a real part and an imaginary part, expressed as a + bi where a and b are real numbers.
- Square root: The value that, when multiplied by itself, gives the original number. For negative numbers, this involves imaginary numbers.
- Approximation: The process of finding a number close to the exact value, often used for non-perfect squares.
- Prime factorization: Breaking down a number into its prime factors, useful for understanding its structure and simplifying calculations.
Explore More algebra
Previous to Square Root of -124
Jaskaran Singh Saluja
About the Author
Jaskaran Singh Saluja is a math wizard with nearly three years of experience as a math teacher. His expertise is in algebra, so he can make algebra classes interesting by turning tricky equations into simple puzzles.
Fun Fact
: He loves to play the quiz with kids through algebra to make kids love it.
