127 LearnersLast updated on May 26th, 2025
Square Root of -98
When a number is squared, the result is a perfect square. The inverse operation is finding the square root. Square roots are used in various fields such as engineering, physics, and mathematics. Here, we will discuss the square root of -98.
What is the Square Root of -98?
The square root is the inverse operation of squaring a number. Since -98 is negative, it does not have a real number square root. The square root of -98 is expressed in terms of imaginary numbers. In radical form, it is expressed as √(-98), or equivalently as √98i, where i is the imaginary unit with the property that i² = -1. The value of √98 is approximately 9.899, so √(-98) = 9.899i.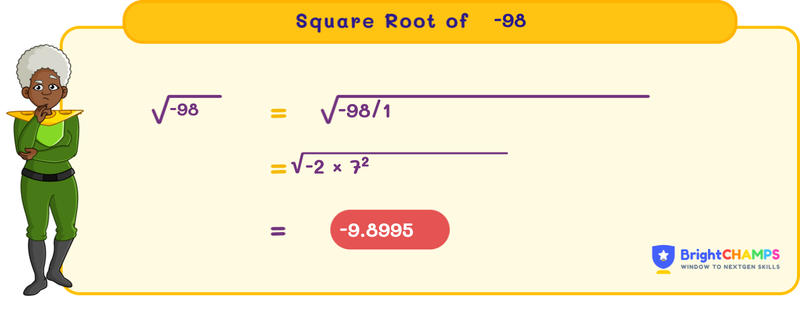
Finding the Square Root of -98
Finding the square root of a negative number involves using the imaginary unit i. We express the square root of a negative number in terms of i and the positive part of the number. For √(-98), we first calculate the square root of 98, and then multiply by i:
1. Calculate √98.
2. Multiply the result by i.
Square Root of -98 by Prime Factorization Method
The prime factorization method is used to simplify the square root of positive numbers. For negative numbers, we factor the positive part. Let’s see how 98 is broken down into its prime factors:
Step 1: Prime factorize 98. 98 = 2 × 7 × 7 = 2 × 7²
Step 2: Use the prime factors to simplify: √98 = √(2 × 7²) = 7√2
Step 3: Multiply by i: The square root of -98 is 7√2i.
Square Root of -98 by Long Division Method
The long division method is not applicable to negative numbers directly for real square roots, but it can be used to approximate the square root of the positive part (98) before multiplying by i.
Step 1: Group digits of 98 from right to left as 98.
Step 2: Find an n such that n² is closest to 98. Here, n = 9 since 9² = 81.
Step 3: The approximate square root of 98 is 9.899.
Step 4: Multiply by i: √(-98) = 9.899i.
Square Root of -98 by Approximation Method
The approximation method can also be used for the positive part of -98.
Step 1: Find the closest perfect squares around 98. The closest perfect squares to 98 are 81 (9²) and 100 (10²).
Step 2: Estimate √98 using these perfect squares. √98 is approximately 9.899.
Step 3: Multiply by i to get the square root of -98: √(-98) = 9.899i.
Common Mistakes and How to Avoid Them in Calculating the Square Root of -98
Mistakes may occur when dealing with negative square roots, especially involving imaginary numbers. Here are a few common errors and how to avoid them.
Mistake 1
Neglecting the Imaginary Unit
A common mistake is ignoring the imaginary unit i when dealing with negative square roots. Remember, the square root of a negative number involves i.
For example, √(-25) should be written as 5i, not just 5.
Square Root of -98 Examples
Problem 1
What is the square root of -196?
The square root is 14i.
Explanation
First, find the square root of 196, which is 14.
Since the original number is negative, we multiply by i, resulting in 14i.
Problem 2
Calculate the square root of -49.
7i
Explanation
Find the square root of 49, which is 7.
Multiply by i for the negative square root: √(-49) = 7i.
Problem 3
What is the result of √(-16) * √(-4)?
-8
Explanation
√(-16) = 4i and √(-4) = 2i.
Multiply: 4i * 2i = 8i².
Since i² = -1, the result is -8.
Problem 4
Find the product of √(-9) and √(-1).
3
Explanation
√(-9) = 3i and √(-1) = i.
Multiply: 3i * i = 3i² = 3(-1) = -3.
Problem 5
If z = √(-64), what is the magnitude of z?
8
Explanation
The magnitude of a complex number a + bi is √(a² + b²). Here, z = 8i, so the magnitude is √(0² + 8²) = √64 = 8.
FAQ on Square Root of -98
1.What is the square root of -98 in simplest form?
2.How do you calculate the square root of -98?
3.What is the imaginary unit i?
4.Can a negative number have a real square root?
5.What is the principal square root of a negative number?
Important Glossaries for the Square Root of -98
- Imaginary unit: The imaginary unit is denoted as i and represents √(-1). It is used to express the square roots of negative numbers.
- Complex number: A number comprising a real part and an imaginary part, expressed as a + bi, where a and b are real numbers.
- Real number: A value representing a quantity along a continuous line, including all rational and irrational numbers.
- Square root: The square root of a number x is a number y such that y² = x. For negative numbers, it involves the imaginary unit.
- Prime factorization: The process of expressing a number as the product of its prime factors, used to simplify square roots of positive numbers.
Explore More algebra
Previous to Square Root of -98
Jaskaran Singh Saluja
About the Author
Jaskaran Singh Saluja is a math wizard with nearly three years of experience as a math teacher. His expertise is in algebra, so he can make algebra classes interesting by turning tricky equations into simple puzzles.
Fun Fact
: He loves to play the quiz with kids through algebra to make kids love it.
