Table Of Contents
130 LearnersLast updated on March 2nd, 2025
MCMXCII Roman Numerals
Roman numerals, developed by the ancient Romans, use a combination of seven symbols — I, V, X, L, C, D, and M to represent numbers. They were essential for recording transactions, keeping track of data, and labeling military units. In this topic, we will explore the Roman numeral MCMXCII.
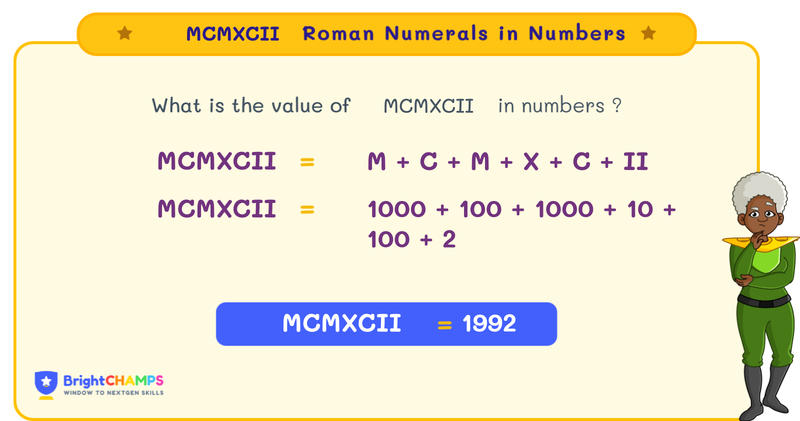
What is MCMXCII in Roman Numerals?
The Roman numeric system was developed by the ancient Romans to simplify counting and was widely used across Europe until the late Middle Ages. It employs seven symbols: I, V, X, L, C, D, and M.
These symbols are combined in various ways to represent different numbers. MCMXCII in Roman numerals can be translated into numeric form by combining the values of each symbol: MCMXCII = 1992.
Let's delve deeper into the Roman numeral MCMXCII, how it is written, common mistakes, and ways to avoid them.
Basic Rules for MCMXCII in Roman Numerals
When writing Roman numerals, several rules should be followed depending on the numeral being written. This section covers the rules for writing Roman numerals and how to represent them.
Rule 1: Addition Method:
If a larger numeral is followed by a smaller one, add them. For example, VI = 5 + 1 = 6.
Rule 2: Repetition Method:
Repeating a symbol up to three times increases its value. For example, CCC = 300.
Rule 3: Subtraction Method:
If a smaller numeral precedes a larger one, subtract the smaller from the larger. For example, IV = 5 - 1 = 4.
Rule 4: Limitation Rule:
Symbols cannot be repeated more than three times, and some symbols, like V, L, and D, cannot be repeated. For example, 10 is written as X, not VV.
How to Write MCMXCII in Roman Numerals?
Let’s learn how to write MCMXCII in Roman numerals using two methods:
- By Expansion Method
- By Grouping Method
MCMXCII in Roman Numerals by Expansion Method
The expansion method involves breaking down Roman numerals into parts and converting them to numerals to get the final number.
Step 1: Break the Roman numerals into parts.
Step 2: Convert each part into its numerical value.
Step 3: Add the values together.
For MCMXCII,
Step 1: Break down the numerals. MCMXCII = M + CM + XC + II
Step 2: Convert each part: M = 1000 CM = 900 XC = 90 II = 2 Step 3: Combine the values: 1000 + 900 + 90 + 2 = 1992 Therefore, MCMXCII is 1992.
MCMXCII in Roman Numerals by Grouping Method
Using subtraction and addition rules, the grouping method simplifies Roman numerals by breaking them into smaller groups.
Step 1: Start with the largest numeral.
Step 2: Apply addition and subtraction rules.
Example: MCMXCII
Step 1: Begin with the largest numerals. The groups are M, CM, XC, and II. M = 1000
Step 2: Add or subtract as needed. Add CM (900), XC (90), and II (2) to M.
Therefore, MCMXCII totals to 1992.
Numbers Related to MCMXCII in Roman Numerals
Roman numerals follow a pattern. Here are numbers related to MCMXCII:
| Roman Numeral | Number | Breakdown |
|---|---|---|
| MCMXC | 1990 | M + CM + XC |
| MCMXCI | 1991 | M + CM + XC + I |
| MCMXCII | 1992 | M + CM + XC + I + I |
| MCMXCIII | 1993 | M + CM + XC + I + I + I |
| MCMXCIV | 1994 | M + CM + XC + IV |
| MCMXCV | 1995 | M + CM + XC + V |
| MCMXCVI | 1996 | M + CM + XC + V + I |
| MCMXCVII | 1997 | M + CM + XC + V + I + I |
| MCMXCVIII | 1998 | M + CM + XC + V + I + I + I |
| MCMXCIX | 1999 | M + CM + XC + IX |
Common Mistakes and How to Avoid Them in MCMXCII Roman Numerals
Students often make mistakes with Roman numerals. Below are some common errors and tips to avoid them.
Mistake 1
Mistakes when applying the repetition method
Beginners may confuse when they can repeat symbols. Remember, symbols like V, L, and D can't be repeated. Writing VV for 10 is incorrect; the correct symbol is X.
MCMXCII Roman Numerals Examples
Problem 1
What is the sum of MCMXCII and VIII? Write the answer in Roman numerals.
The sum is MM.
Explanation
Convert both Roman numerals into their decimal form:
MCMXCII = 1992
VIII = 8
Now add both numbers: 1992 + 8 = 2000
Convert the number into its Roman numeral: 2000 = MM
Problem 2
Calculate the difference between MM - MCMXCII. Write in Roman numerals.
The difference is VIII.
Explanation
Convert the Roman numerals into their decimal form:
MM = 2000
MCMXCII = 1992
Now subtract the numbers: 2000 - 1992 = 8
Convert the number into its Roman numeral: 8 = VIII
Problem 3
Divide MCMXCII by IV and write the answer in Roman numerals.
D.
Explanation
Convert MCMXCII into its decimal form:
MCMXCII = 1992
Divide by 4: 1992 / 4 = 498
Write 498 in Roman numerals: 400 (CD) + 90 (XC) + 8 (VIII) = CDXCVIII
Problem 4
What is the product of MCMXCII and III?
The product is MMMMDCCLXXVI.
Explanation
Write MCMXCII and III in numbers:
MCMXCII = 1992
III = 3
Multiply the numbers: 1992 × 3 = 5976
Convert 5976 into its Roman numerals: 5000 (MMMMM) + 900 (CM) + 70 (LXX) + 6 (VI) = MMMMDCCLXXVI
Problem 5
Convert MCMXCII into its decimal form.
In decimal form, MCMXCII is 1992.
Explanation
Break MCMXCII into components:
M = 1000
CM = 900 (1000 - 100)
XC = 90 (100 - 10)
II = 2 (I + I)
Add values: 1000 + 900 + 90 + 2 = 1992
FAQs on MCMXCII in Roman Numerals
1.What is CXCII in Roman numerals?
2.Is MCMXCII a prime number?
3.What is MCMXCII + MCMXCII?
4.What is MMII?
5.Subtract XCII from MCMXCII
Important Glossaries for MCMXCII in Roman Numerals
- Addition Method: Adding numerals when a larger numeral precedes a smaller one.
- Subtraction Method: Subtracting a smaller numeral from a larger one when it precedes it.
- Repetition Method: Repeating numerals up to three times to increase value.
- Place Value: The value represented by a numeral's position.
- Grouping Method: Simplifying numerals by organizing them into smaller groups.
Explore More numbers
Previous to MCMXCII Roman Numerals
Hiralee Lalitkumar Makwana
About the Author
Hiralee Lalitkumar Makwana has almost two years of teaching experience. She is a number ninja as she loves numbers. Her interest in numbers can be seen in the way she cracks math puzzles and hidden patterns.
Fun Fact
: She loves to read number jokes and games.
