Factors of 216

The factors of 216 are -  

1,2,3,4,6,8,9,12,18,24,27,36,54,72,108,216.

Negative factors of 216: (-1,-216), (-2,-108), (-3,-72), (-4,-54), (-6,-36), (-8,-27), (-9,-24), (-12,-18).

Prime factors of 216: 2×2×2×3×3×3

Prime Factorization of 216: 2×2×2×3×3×3 = 2³×3³

The sum of Factors of 216: 1+2+3+4+6+8+9+12+18+24+27+36+54+72+108+216 = 600

We can find factors of 216 in some most easy ways, mentioned below:

 

• Multiplication Method

 

• Division Method

 

• Prime Factor and Prime Factorization

 

• Factor Tree
   

This particular method usually finds the pair of factors which, on multiplication together, produces 216. Let us find the pairs which, on multiplication, yields 216.

1×216=216

2×108=216

3×72=216

4×54=216

6×36=216

8×27=216

9×24=216

12×18=216

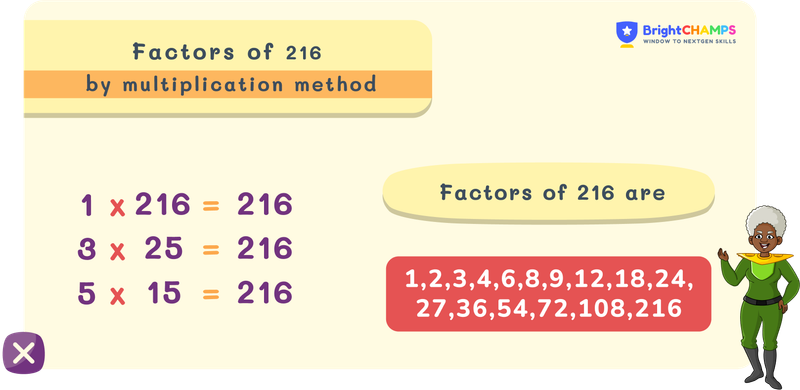
So, we got the factors as 1,2,3,4,6,8,9,12,18,24,27,36,54,72,108,216.

The division method finds the numbers that perfectly divides the given number 216. To find the factors of 216, we have to divide 216 by all possible natural numbers less than 216.

1,2,3,4,6,8,9,12,18,24,27,36,54,72,108,216 are the only factors that the number 216 has. So to verify the factors of 216 using the division method, we just need to divide 216 by each factor.

216/1 =216

216/2 =108

216/3=72

216/4=54

216/6=36

216/9=24

216/12=18

TASK: Do the same as above with the remaining factors.
 

To get the 216 as their product, we multiply a set of prime numbers, and that set is called prime factors. Prime factorization is the process of breaking down the number into its prime factors.

Prime Factors of 216: 2,3.

Prime Factorization of 261: 2×2×2×3×3×3 = 2³×3³

 

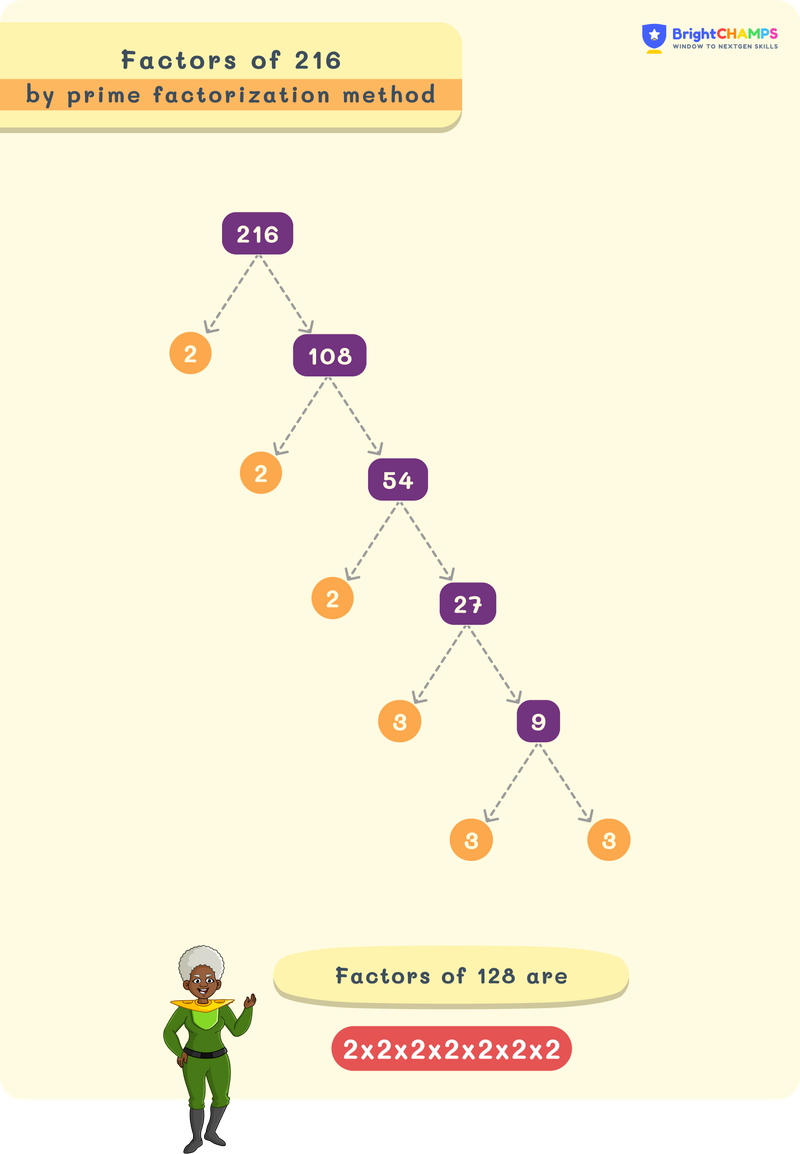
Factor tree: The number 216 is written on top and two branches are drawn.

Fill in the branches with a factor pair of the number above, i.e., 216.

Continue this process until each branch ends with a prime factor (number).

The first two branches of the factor tree of 216 are 2 and 108, then proceeding to 108, we get 2 and 54.
Similarly, for 54: 2 and 27

For 27: 3 and 9

For 9: 3 and 3

 

Factor Pairs

Positive pair factors:  (1,216), (2,108), (3,72), (4,54), (6,36), (8,27), (9,24), (12,18).

Negative pair factors: (-1,-216), (-2,-108), (-3,-72), (-4,-54), (-6,-36), (-8,-27), (-9,-24), (-12,-18).
 

• Factors - These are numbers that divide the given number without leaving any remainder or the remainder as 0.

 

• Prime Factorization - It involves factoring the number into its prime factors.

 

• Prime factors - These are the prime numbers which on multiplication together results into the original number whose prime factors are to be obtained.

 

• Composite numbers - These are numbers having more than two factors.

 

• Multiple - It is a product of the given number and any other integer.