Summarize this article:
 181 Learners
181 LearnersLast updated on August 5, 2025
Square Root of -135

When a number is multiplied by itself, the result is its square. The opposite operation is the square root. Square roots are used in many fields, including engineering and finance. Here, we will discuss the square root of -135.

What is the Square Root of -135?
The square root is the inverse operation of squaring a number. Since -135 is a negative number, its square root involves imaginary numbers. The square root of -135 is expressed in terms of the imaginary unit 'i' as √(-135) = √(135) * i. The value of √(135) is approximately 11.61895, making the square root of -135 approximately 11.61895i, which is a complex number.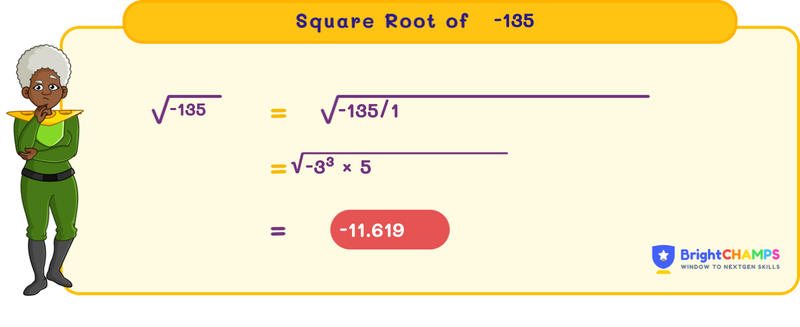

Understanding the Concept of Square Root of -135
For negative numbers like -135, the square root involves imaginary numbers. This is because no real number squared will result in a negative number. Therefore, we use 'i' to denote the imaginary unit where i² = -1. This allows us to express the square root of negative numbers as complex numbers. For example, the square root of -135 is written as √(-135) = √135 * i.

Breaking Down the Components of the Square Root of -135
To understand the square root of -135, we first find the square root of 135, which is a positive number. The prime factorization of 135 is 3 x 3 x 3 x 5, which simplifies as √135 = 3√15. Since -135 is negative, we multiply this by 'i', giving us 3√15 * i or approximately 11.61895i.

Square Root of -135 in Practical Scenarios
While the square root of negative numbers does not have direct applications in real-world measurements, it is essential in fields involving complex numbers, such as electrical engineering and quantum mechanics. The square root of -135 can be used to solve equations in these fields where complex numbers naturally arise.

Interpreting the Square Root of -135
The square root of -135 is a complex number, which can be visualized on the complex plane. The real part is 0, and the imaginary part is approximately 11.61895. In polar coordinates, this is expressed with a magnitude of 11.61895 and an angle of 90 degrees (or π/2 radians) from the positive real axis.

Common Mistakes and How to Avoid Them in Understanding Square Roots of Negative Numbers
Students often make errors when dealing with square roots of negative numbers, such as misunderstanding the role of the imaginary unit 'i' or confusing square roots with other operations. Let's look at some common mistakes and how to avoid them.

Square Root of -135 Examples

Problem 1
If the impedance of an electrical circuit is represented as √(-135) ohms, what is its magnitude in ohms?

The magnitude is approximately 11.61895 ohms.
Explanation
The magnitude of a complex number is given by the modulus.
For √(-135), the magnitude is √135, which is approximately 11.61895.

Problem 2
A complex number is given as 3√15i in a circuit. What is the square root of -135 expressed in this form?

The square root is 3√15i.
Explanation
The square root of -135 is 3√15i, derived from the prime factorization of 135, which is 3 x 3 x 3 x 5, simplifying to 3√15. Multiplying by 'i' gives us 3√15i.

Problem 3
Calculate the magnitude of 5 times the square root of -135.

The magnitude is approximately 58.09475.
Explanation
First, find the magnitude of √(-135), which is √135 ≈ 11.61895.
Then, multiply by 5: 11.61895 * 5 = 58.09475.

Problem 4
What does the expression √(-135) * √(-1) simplify to?

The expression simplifies to 135.
Explanation
The expression √(-135) * √(-1) becomes √(135) * i * i = 135, since i² = -1.

Problem 5
If the square root of -135 is used in a quantum mechanics calculation, what is its significance?

It represents a complex amplitude or state.
Explanation
In quantum mechanics, complex numbers are used to represent probabilities and wave functions.
The square root of -135 would represent a state with an imaginary component.


FAQ on Square Root of -135
1.What is √(-135) in terms of 'i'?
2.What is the magnitude of √(-135)?
3.Can the square root of -135 be a real number?
4.What is the principal square root of -135?
5.How is the square root of -135 used in electrical engineering?
6.How does learning Algebra help students in Canada make better decisions in daily life?
7.How can cultural or local activities in Canada support learning Algebra topics such as Square Root of -135?
8.How do technology and digital tools in Canada support learning Algebra and Square Root of -135?
9.Does learning Algebra support future career opportunities for students in Canada?

Important Glossaries for the Square Root of -135
- Imaginary Number: An imaginary number is a number that can be written as a real number multiplied by the imaginary unit 'i', where i² = -1.
- Complex Number: A complex number consists of a real part and an imaginary part, expressed as a + bi, where 'a' and 'b' are real numbers.
- Magnitude: The magnitude (or modulus) of a complex number is the distance from the origin to the point represented by the number in the complex plane.
- Principal Square Root: The principal square root of a negative number is the positive imaginary number derived from its square root calculation.
- Prime Factorization: Prime factorization is expressing a number as a product of its prime factors, useful for simplifying square roots.

Explore More algebra
![Important Math Links Icon]() Previous to Square Root of -135
Previous to Square Root of -135
![Important Math Links Icon]() Next to Square Root of -135
Next to Square Root of -135

About BrightChamps in Canada


Jaskaran Singh Saluja
About the Author
Jaskaran Singh Saluja is a math wizard with nearly three years of experience as a math teacher. His expertise is in algebra, so he can make algebra classes interesting by turning tricky equations into simple puzzles.
Fun Fact
: He loves to play the quiz with kids through algebra to make kids love it.




