Summarize this article:
 192 Learners
192 LearnersLast updated on 5 August 2025
Square Root of 1828

If a number is multiplied by the same number, the result is a square. The inverse of the square is a square root. The square root is used in fields such as vehicle design, finance, etc. Here, we will discuss the square root of 1828.

What is the Square Root of 1828?
The square root is the inverse of squaring a number. 1828 is not a perfect square. The square root of 1828 can be expressed in both radical and exponential forms. In radical form, it is expressed as √1828, whereas in exponential form as (1828)^(1/2). √1828 ≈ 42.7465, which is an irrational number because it cannot be expressed as a fraction of two integers.
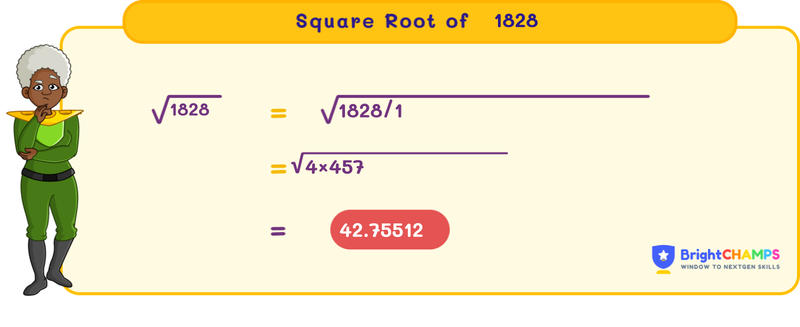

Finding the Square Root of 1828
The prime factorization method is used for perfect square numbers. For non-perfect square numbers, methods like long division and approximation are used. Let us now learn these methods:
- Prime factorization method
- Long division method
- Approximation method

Square Root of 1828 by Prime Factorization Method
The product of prime factors is the prime factorization of a number. Now let us look at how 1828 is broken down into its prime factors:
Step 1: Finding the prime factors of 1828 Breaking it down, we get 2 x 2 x 457: 2^2 x 457
Step 2: Now we found out the prime factors of 1828. Since 1828 is not a perfect square, the digits of the number cannot be grouped in pairs.
Therefore, calculating the square root of 1828 using prime factorization directly is not possible.

Square Root of 1828 by Long Division Method
The long division method is particularly useful for non-perfect square numbers. In this method, we should check the closest perfect square number for the given number. Let us now learn how to find the square root using the long division method, step by step.
Step 1: To begin with, group the numbers from right to left. In the case of 1828, group it as 18 and 28.
Step 2: Find n whose square is less than or equal to 18. We can say n is ‘4’ because 4 x 4 = 16, which is less than 18. The quotient is 4, and after subtracting 16 from 18, the remainder is 2.
Step 3: Bring down 28, creating a new dividend of 228. Add the old divisor with itself: 4 + 4 = 8, which will be our new divisor.
Step 4: Find n such that 8n x n is less than or equal to 228. Let n be 2, then 82 x 2 = 164.
Step 5: Subtract 164 from 228, resulting in 64. The quotient is 42.
Step 6: Since the dividend is less than the divisor, add a decimal point and bring down two zeros. The new dividend is 6400.
Step 7: Find the new divisor. Adding another 2 to 84 gives 842. Now find n such that 842n x n is less than or equal to 6400. Let n be 7, then 842 x 7 = 5894.
Step 8: Subtract 5894 from 6400, resulting in 506.
Step 9: The quotient is approximately 42.7.
Step 10: Continue these steps until you achieve the desired decimal precision.
Thus, the square root of √1828 is approximately 42.7465.

Square Root of 1828 by Approximation Method
The approximation method is another approach for finding square roots, providing an easy way to estimate the square root of a given number. Let us learn how to find the square root of 1828 using the approximation method.
Step 1: Identify the closest perfect squares around 1828. The smallest perfect square is 1764 (42^2) and the largest is 1849 (43^2). √1828 falls between 42 and 43.
Step 2: Apply the formula: (Given number - smallest perfect square) / (Greater perfect square - smallest perfect square)
Using the formula: (1828 - 1764) / (1849 - 1764) = 64 / 85 ≈ 0.7529
Adding this decimal to the smaller square root: 42 + 0.7529 ≈ 42.7529
Therefore, the square root of 1828 is approximately 42.7529.

Common Mistakes and How to Avoid Them in the Square Root of 1828
Students often make mistakes while finding the square root, such as neglecting the negative square root or skipping steps in the long division method. Let us discuss a few common mistakes in detail.

Square Root of 1828 Examples

Problem 1
Can you help Max find the area of a square box if its side length is given as √328?

The area of the square is approximately 328 square units.
Explanation
The area of a square = side^2.
The side length is given as √328.
Area = side^2 = √328 x √328 = 18.11 x 18.11 ≈ 328.
Therefore, the area of the square is approximately 328 square units.

Problem 2
A square-shaped building measuring 1828 square feet is built; if each of the sides is √1828, what will be the square feet of half of the building?

914 square feet
Explanation
To find half the area of the square-shaped building, divide the total area by 2.
Dividing 1828 by 2 = 914 So half of the building measures 914 square feet.

Problem 3
Calculate √1828 x 5.

Approximately 213.73
Explanation
First, find the square root of 1828, which is approximately 42.7465.
Then multiply 42.7465 by 5.
So, 42.7465 x 5 ≈ 213.73

Problem 4
What will be the square root of (828 + 1000)?

The square root is approximately 42.7
Explanation
To find the square root, first find the sum of (828 + 1000).
828 + 1000 = 1828, and then √1828 ≈ 42.7.
Therefore, the square root of (828 + 1000) is approximately ±42.7.

Problem 5
Find the perimeter of the rectangle if its length ‘l’ is √328 units and the width ‘w’ is 38 units.

The perimeter of the rectangle is approximately 113.22 units.
Explanation
Perimeter of a rectangle = 2 × (length + width)
Perimeter = 2 × (√328 + 38) = 2 × (18.11 + 38) = 2 × 56.11 ≈ 113.22 units.


FAQ on Square Root of 1828
1.What is √1828 in its simplest form?
2.Mention the factors of 1828.
3.Calculate the square of 1828.
4.Is 1828 a prime number?
5.1828 is divisible by?
6.How does learning Algebra help students in India make better decisions in daily life?
7.How can cultural or local activities in India support learning Algebra topics such as Square Root of 1828?
8.How do technology and digital tools in India support learning Algebra and Square Root of 1828?
9.Does learning Algebra support future career opportunities for students in India?

Important Glossaries for the Square Root of 1828
- Square root: A square root is the inverse operation of squaring a number. For example, 4^2 = 16, and the inverse is √16 = 4.
- Irrational number: An irrational number cannot be expressed as a fraction of two integers, where the denominator is not zero.
- Approximation method: A technique used to estimate the value of a square root by identifying nearby perfect squares and using interpolation.
- Long division method: A step-by-step division process used to find the square root of non-perfect squares.
- Prime factorization: Breaking down a number into its prime factors, which are used to determine properties like square roots.

Explore More algebra
![Important Math Links Icon]() Previous to Square Root of 1828
Previous to Square Root of 1828
![Important Math Links Icon]() Next to Square Root of 1828
Next to Square Root of 1828

About BrightChamps in India


Jaskaran Singh Saluja
About the Author
Jaskaran Singh Saluja is a math wizard with nearly three years of experience as a math teacher. His expertise is in algebra, so he can make algebra classes interesting by turning tricky equations into simple puzzles.
Fun Fact
: He loves to play the quiz with kids through algebra to make kids love it.




