Summarize this article:
 987 Learners
987 LearnersLast updated on 5 August 2025
Factors of 63

Factors of 63 are the whole numbers that can divide the number evenly without the remainder. In daily life, unknowingly, we apply factors to divide anything evenly in the group. In this article, we will study different methods to solve and solved examples of factors of 63.

What are the Factors of 63?
The factors of 63 are 1, 3, 7, 9, 21 and 63
Negative Factors
These are negative counterparts of the positive factors.
Negative factors: -1, -3, -7, -9, -21, -63
Prime Factors
Prime factors are the prime numbers themselves, when multiplied together, give 63 as the product.
Prime factors: 3, 7
Prime Factorization
Prime factorization involves breaking 63 into its prime factors
It is expressed as 32 × 71
Table listing the factors of 63
|
Positive Factors |
1, 3, 7, 9, 21, 63 |
|
Negative Factors |
-1, -3, -7, -9, -21, -63 |
|
Prime Factors |
2, 7 |
|
Prime Factorization |
32 × 71 |

How to Find the Factors of 63?
There are different methods to find the factors of 63.
Methods to find the factors of 63:
- Multiplication Method
- Division Method
- Prime Factor and Prime Factorization
- Factor Tree

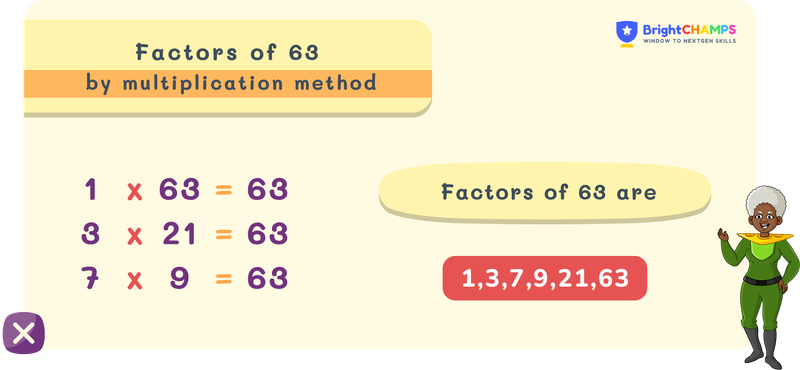
Finding Factors Using Multiplication Method
The multiplication method finds the pair of factors that give 63 as their product.
Step-by-step process
Step 1: Find the pair of numbers whose product is 63.
Step 2: The factors are those numbers, when multiplied, give 63.
Step 3: Make a list of numbers whose product will be 63.
A list of numbers whose products are 63 is given below:
1 × 63 = 63
3 × 21 = 63
7 × 9 = 63

Finding Factors Using Division Method
The division method finds the numbers that fully divide the given number.
Step-by-step process:
Step 1: Since every number is divisible by 1, 1 will always be a factor. Example: 63÷1 = 63
Step 2: Move to the next integer. Both divisor and quotient are the factors.
Picture showing the division method:


Prime Factors and Prime Factorization
Multiplying prime numbers to get the given number as their product is called prime factors. Prime factorization is breaking down the number into its prime factors.
Prime Factors of 63
Number 63 has only one prime factor.
Prime factors of 63: 3, 7
To find the prime factors of 63, divide 63 with the prime numbers 3 and 7.
- 63÷3 = 21
- 21÷3 = 7
- 7÷7 = 1
Prime Factorization of 63:
Prime Factorization breaks down the prime factors of 63
Expressed as 32 × 71

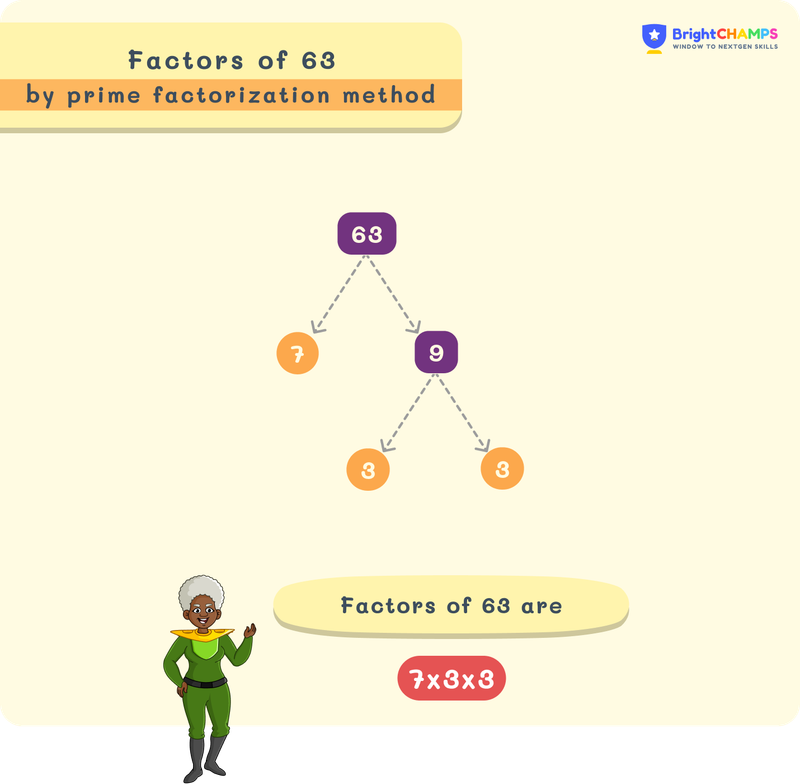
Factor Tree
The prime factorization is visually represented using the factor tree. It helps to understand the process easily. In this factor tree, each branch splits into prime factors.
Factor Tree for 63:
Factors of 63 can be written in both positive pairs and negative pairs. They are like team members. Their product will be equal to the number given.
Positive Factor Pairs: (1,63), (3,21), (7,9)
Negative Factor Pairs: (-1,-63), (-3,-21), (-7,-9)

Common Mistakes and How to Avoid Them in Factors of 63
Mistakes can occur while finding the factors. Learn about the common errors that can occur. Solutions to solve the common mistakes are given below.

Factors of 63 examples

Problem 1
If we add all the factors of 63, what will be the sum?

The sum of factors is 104
Explanation
Add all the factors of 63 (1+3+7+9+21+63) to get the sum 104.

Problem 2
Find the sum of the factor pair (7,9) and verify whether the sum is divisible by 2.

The sum of the factor pair (7,9) is 16 and the sum is divisible by 2
Explanation
When the factor pair (7,9) is added (7+9) we get 16 as the sum. To verify whether 16 is divisible by 2, we check if the
remainder is zero when 16 is divided by 2. Here, the remainder is zero. Hence, 16 is divisible by 2.

Problem 3
How many single-digit factors are there for 63?

There are four single-digit factors.
Explanation
Write down the factors 63 and identify the single-digit factors.
The single-digit factors are 1, 3, 7 and 9.


FAQs on Factors of 63
1.What are the two prime factors of 63?
2.Is 63 a factor of 4?
3.What is the HCF of 27 and 63?
4.Is 7 a factor of 63?
5.What is the GCF of 63 and 81?
6.How can children in Indonesia use numbers in everyday life to understand Factors of 63?
7.What are some fun ways kids in Indonesia can practice Factors of 63 with numbers?
8.What role do numbers and Factors of 63 play in helping children in Indonesia develop problem-solving skills?
9.How can families in Indonesia create number-rich environments to improve Factors of 63 skills?

Glossary
Whole Number: Numbers starting from zero.
Factors: Numbers that divide the given number, leaving zero as the remainder.
Prime Factors: Prime numbers that multiply together to form the given number.
Prime Factorization: Process of breaking down the prime factors.
GCF: Greatest Common Factor is the largest possible number seen in two or more numbers.

Explore More numbers
![Important Math Links Icon]() Previous to Factors of 63
Previous to Factors of 63
![Important Math Links Icon]() Next to Factors of 63
Next to Factors of 63

About BrightChamps in Indonesia


Hiralee Lalitkumar Makwana
About the Author
Hiralee Lalitkumar Makwana has almost two years of teaching experience. She is a number ninja as she loves numbers. Her interest in numbers can be seen in the way she cracks math puzzles and hidden patterns.
Fun Fact
: She loves to read number jokes and games.




