212 LearnersLast updated on May 26th, 2025
Factors of 338
Learn how to find the Factors of 338 with step-by-step methods like Prime Factorization and more, with examples. Perfect for math lovers!
What are the Factors of 338
The factors of 338 are the numbers that divide 338 evenly.
Positive Factors: These are the positive numbers that divide 338 evenly.
Positive factors are 1, 2, 13, 26, 169, and 338.
Negative Factors: These are negative counterparts of the positive factors.
Negative factors are -1, -2, -13, -26, -169, -338
Prime Factors: Prime factors are the prime numbers themselves, when multiplied together, give 338 as the product.
Prime factors: 2, 13
Prime Factorization: Prime factorization involves breaking 338 into its prime factors.
It is expressed as 21×132
Table listing the factors of 338
|
Positive Factors |
1, 2, 13, 26, 169, 338 |
|
Negative Factors |
-1, -2, -13, -26, -169, -338 |
|
Prime Factors |
2, 13 |
|
Prime Factorization |
21×132 |
This breakdown helps in understanding the various factors of 338, whether they are positive or negative, as well as how prime factorization works for this number.
How to Find the Factors of 338?
There are different methods to find the factors of 338.
Methods to find the factors of 338:
- Multiplication Method
- Division Method
- Prime Factor and Prime Factorization
- Factor Tree
Finding Factors Using Multiplication Method
The multiplication method finds the pair of factors that give 338 as their product.
Step 1: Find the pair of numbers whose product is 338.
Step 2: The factors are those numbers, when multiplied, give 338.
Step 3: Make a list of numbers whose product will be 338.
A list of numbers whose products are 338 is given below:
- 1 × 338 = 338
- 2 × 169 = 338
- 13 × 26 = 338
Thus, the factors of 338 are 1, 2, 13, 26, 169, and 338.
Finding Factors Using Division Method
The division method finds the numbers that fully divide the given number. The steps are given below:
Step 1: Since every number is divisible by 1, 1 will always be a factor.
Example: 338÷1=338
Step 2: Move to the next integer. The factors of the number include the number that is used to divide and the number of times the particular number is divided.
Thus, the factors of 338 are 1, 2, 13, 26, 169, and 338.
Prime Factors and Prime Factorization
Multiplying prime numbers to get the given number as their product is called prime factors. A number, when simplified using its factors and expressed in the form of prime factors, is the prime factorization of a number.
Prime Factors of 338: Number 338 has two prime factors.
Prime factors of 338: 2, 13
Steps to Find the Prime Factors of 338:
Divide 338 by the smallest prime number 2:
- 338 ÷ 2 = 169
Divide 169 by the next prime number 13:
- 169 ÷ 13 = 13
Divide 13 by itself:
- 13 ÷ 13 = 1
Prime Factorization of 338:
Expressed as 21×132
Factor Tree
The prime factorization is visually represented using the factor tree. It helps to understand the process easily. In this factor tree, each branch splits into prime factors.
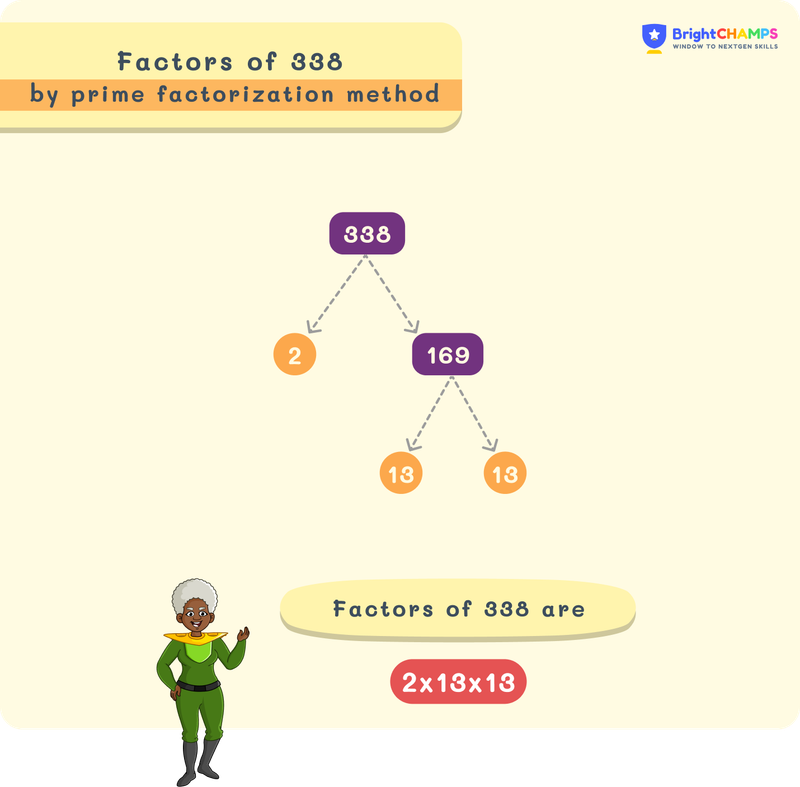
This tree shows the breakdown of 338 into its prime factors: 2 × 13 × 13.
Positive and Negative Factor Pairs of 338
Factors of 338 can be written in both positive pairs and negative pairs. They are like team members. Their product will be equal to the number given.
Positive Factor Pairs: (1, 338), (2, 169), (13, 26)
Negative Factor Pairs: (-1, -338), (-2, -169), (-13, -26)
Common Mistakes and How to Avoid Them in Factors of 338
Mistakes can occur while finding the factors. Learn about the common errors that can occur. Solutions to solve the common mistakes are given below.
Mistake 1
Assuming 13 as the only factor of 338
Since numbers divisible by 13 are sometimes assumed to have no other factors, remember to check for all divisors. Other numbers that can divide 338 completely are 1, 2, 26, 169, and 338.
Solved Examples for Factors of 338
Problem 1
Can you check whether 388 and 32 are co-prime?
No, 388 and 32 are not co-prime.
Explanation
To check whether two numbers are co-prime, list their factors first.
Once you have listed the factors, identify the common factors and determine the GCF.
If the GCF is greater than 1, then the numbers are not co-prime.
- Factors of 388: 1, 2, 4, 97, 194, 388
- Factors of 32: 1, 2, 4, 8, 16, 32
Here, the GCF is 4. So, 388 and 32 are not co-prime.
For co-prime, the GCF of numbers should be 1.
Problem 2
Verify whether 388 is a multiple of 5
No, 388 is not a multiple of 5.
Explanation
Multiples of 5 are numbers that end in 0 or 5. Since 388 does not end in 0 or 5, it is not a multiple of 5.
Problem 3
Identify the perfect square from the factors of 388
The perfect square factor of 388 is 4, and the root is 2.
Explanation
A perfect square is a number we get when the same number is multiplied twice. When 2 is multiplied twice (2×2), we get the perfect square 4.
Problem 4
Is 388 an even or odd number?
388 is an even number.
Explanation
An even number is divisible by 2 without a remainder. Since 388 ÷ 2 = 194 with no remainder, it is an even number.
Problem 5
Are all factors of 388 divisible by 2?
No, not all factors of 388 are divisible by 2.
Explanation
While 388 is an even number, not all of its factors are even. For example, 97 is a factor of 388 and is not divisible by 2.
FAQ’s for Factors of 388
1.What are the factors of 338?
2.How do you determine if a number is a factor of 338?
3.What is the smallest factor of 338?
4.What is the largest factor of 338?
5.How many factors does 338 have?
6.How many odd factors does 338 have?
7.What factors go into 338?
8.Do any perfect squares exist in the factors of 338?
Glossaries for Factors of 338
- Factor: A number that divides another number completely without leaving a remainder.
- Prime Factorization: The process of breaking down a number into its prime factors and expressing it as a product of prime numbers.
- Negative Factor: The negative counterparts of positive factors. For example, the negative factors of 338 are -1, -2, -13, -26, -169, and -338.
- Factor Tree: A visual representation that breaks down a number into its prime factors step by step, resembling the branches of a tree.
- Multiplication Method: A method of finding factors by identifying pairs of numbers whose product equals the given number.
Explore More numbers
Previous to Factors of 338
Hiralee Lalitkumar Makwana
About the Author
Hiralee Lalitkumar Makwana has almost two years of teaching experience. She is a number ninja as she loves numbers. Her interest in numbers can be seen in the way she cracks math puzzles and hidden patterns.
Fun Fact
: She loves to read number jokes and games.
