Cube Root of 8451

We have learned the definition of the cube root. Now, let’s learn how it is represented using a symbol and exponent. The symbol we use to express the cube root is the radical sign (∛), and the exponent we use is ⅓.

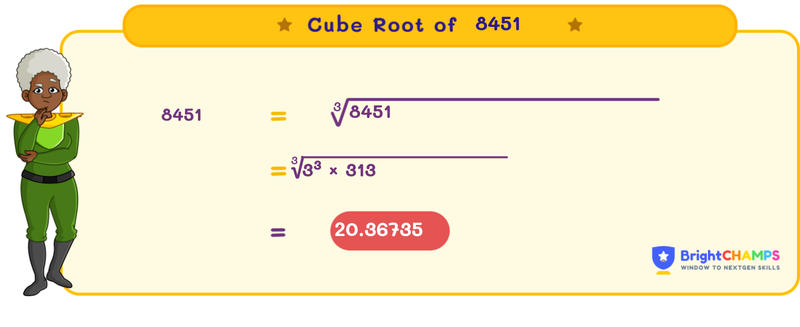
In exponential form, ∛8451 is written as 8451^(1/3). The cube root is just the opposite operation of finding the cube of a number. For example: Assume ‘y’ as the cube root of 8451, then y³ can be 8451. Since the cube root of 8451 is not an exact value, so we can write it as approximately 20.4787.

Finding the cube root of a number is to identify the number that must be multiplied three times resulting in the target number. Now, we will go through the different ways to find the cube root of 8451. The common methods we follow to find the cube root are given below:

• Prime factorization method
• Approximation method
• Subtraction method
• Halley’s method

To find the cube root of a non-perfect number, we often follow Halley’s method. Since 8451 is not a perfect cube, we use Halley’s method.

Let's find the cube root of 8451 using Halley’s method.

The formula is ∛a ≅ x((x^3 + 2a) / (2x^3 + a))

where: a = the number for which the cube root is being calculated

x = the nearest perfect cube

Substituting, a = 8451;

x = 20

∛a ≅ 20((20^3 + 2 × 8451) / (2 × 20^3 + 8451))

∛8451 ≅ 20((8000 + 16902) / (16000 + 8451))

∛8451 ≅ 20.4787

The cube root of 8451 is approximately 20.4787.

• Cube root: The number that is multiplied three times by itself to get the given number is the cube root of that number.
   
• Perfect cube: A number is a perfect cube when it is the product of multiplying a number three times by itself. A perfect cube always results in a whole number. For example: 2 × 2 × 2 = 8, therefore, 8 is a perfect cube.
   
• Exponent: The exponent form of the number denotes the number of times a number can be multiplied by itself. In a^(1/3), ⅓ is the exponent which denotes the cube root of a.
   
• Radical sign: The symbol that is used to represent a root which is expressed as (∛).
   
• Irrational number: The numbers that cannot be put in fractional forms are irrational. For example, the cube root of 8451 is irrational because its decimal form goes on continuously without repeating the numbers.